Basic Problems of Map Projection
The Concept of Map Projection
In mathematics, the meaning of project to establish a one-to-one mapping relationship between two sets of points. Similarly, in cartography, map projection refers to the establishment of one-to-one correspondence between points on the earth’s surface and points on the projection plane. The basic problem of map projection is to use certain mathematical rules to express the longitude and latitude network on the surface of the earth on the plane. For geographic information systems, map projection must be accounted. The use of map projection guarantees the geographical connection and integrity of spatial information. In the process of establishing various geographic information systems, choosing appropriate map projection system is the first consideration. Because the surface of the ellipsoid of the earth is a surface, and the map is usually drawn on a planar drawing, the surface should first be expanded into a plane. However, the sphere is an unfoldable surface, that is, when it is directly expanded into a plane, it is impossible to avoid breaking or folding. It is obviously impractical to draw a map with such a plane with rupture or fold, so a special method must be used to unfold the surface to make it a plane without rupture or fold。
Deformation of Map Projection
Types of Deformation
There are many methods of map projection, and different methods of projection have different forms of longitude and latitude lines. Using map projection method, the sphere can be expanded into a plane. Although it can maintain the integrity and continuity of the figure, they are not completely similar to the shape of the longitude and latitude network on the sphere. This shows that after projection, the longitude and latitude line network on the map has been deformed, so the various ground objects displayed on the map according to geographical coordinates will inevitably be deformed accordingly. This deformation destroys the geometric characteristics (length, direction and area) of the ground objects. Comparing the latitude-longitude network on the map with that on the globe, it can be found that the deformation is manifested in three aspects: length, area and angle. The length and area deformations in projection can be shown by the change of length ratio and area ratio respectively. If the deformation of length or area is zero, there is no deformation of length or area. Angular deformation is the difference between the angle value after projection of an angle and its inherent angle value on the earth’s surface。
1) Length Deformation
That is, the length of longitude and latitude on the map is not exactly the same as that on the globe. The length of longitude and latitude on the map is not all reduced in the same proportion, which indicates that the map has length deformation。
The lengths of longitudes and latitudes on the globe have the following characteristics: first, the lengths of the latitudes vary, with the longest equator, the higher latitude, the shorter latitude, and the zero length of polar latitudes; secondly, on the same latitude, the arcs of latitudes with the same longitude difference are equal; thirdly, all longitudes are equal in length. The deformation of length varies with projection. On the same projection, the length deformation varies not only with the location, but also with the direction at the same point。
2)Area Deformation
That is, because the area of the latitude and longitude grids on the map is different from that of the geodesy, the area of the latitude and longitude grids on the map is not reduced in the same proportion, which indicates that there is an area distortion on the map。
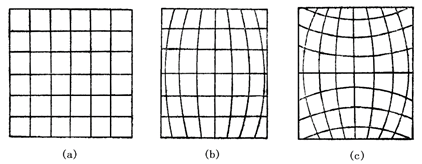
The area of the longitude and latitude grids on the globe has the following characteristics: first, in the same latitude zone, the area of the network with the same longitude difference is equal. Second, in the same longitude zone, the higher the latitude, the smaller the network area. This is not exactly the case on maps. For example, in Figure 4-9-a, in the same latitude zone, the grid areas with equal latitude differences are equal, and these areas are not reduced in the same proportion. The higher the latitude, the larger the area ratio. In Fig. 4-9-b, the grid area with the same longitude difference varies in the same latitude zone, which indicates that the area ratio varies with the longitude. Because the area of the latitude and longitude grids on the map is different from that on the globe, the area of the latitude and longitude grids on the map is not reduced in the same proportion, which indicates that there is an area distortion on the map. Area deformation varies with projection. On the same projection, the area deformation varies with the location。
3) Angular Deformation
It means that the two angles on the map are not equal to the corresponding angles on the sphere. For example, in Figures 4-9-b and 4-9-c, only the central longitude and latitudes intersect at right angles, while the other longitudes and latitudes do not intersect at right angles, while on the globe, both longitudes and latitudes intersect at right angles, which indicates that the angle on the map is deformed. Angular deformation varies with projection. On the same projection map, the angular deformation varies with the location。
The deformation of map projection varies with the change of location, so it is difficult to say in general what deformation it has and how large the deformation is on a map。
Deformed ellipse
Deformation ellipse is a geometric figure showing deformation. As can be seen from Figure 4-9, the same size of longitude and latitude lines on the projection surface are transformed into different shapes and sizes (compared with the three grids in Figure 4-9). In practice, the deformations of each projection are different. By examining the shape and size of the deformed ellipse, which is the representation of a tiny circle on the earth’s surface (called differential circle) in the projection, the difference of the deformations in the projection can be reflected (Fig. 4-10)。
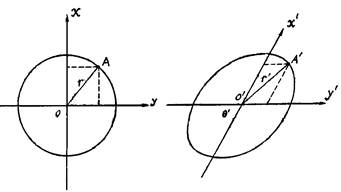
Fig. 51 Differential circles represent projection deformations
Classification of Map Projection
There are many kinds of map projection, which should be classified for the convenience of study and research. the classification methods are different because of the different marks of classification, From the point of view of using maps, we need to understand the following categories。
Classification by deformation property
According to the deformation property map projection can be classified into three categories : isogonal projection, isoproduct projection and arbitrary projection。
1)Equiangular Projection
It is defined that the angle projection composed of two differential lines at any point remains unchanged before and after projection, that is, the corresponding differential area before and after projection remains similar to the figure, so it can be called orthomorphic projection. The angle between any two directions of a point on the projection plane is equal to the angle between the corresponding two lines on the ellipsoid, that is, the angle deformation is zero. The length ratio of equiangular projection in any direction is equal at one point, but the length ratio is different at different locations, that is, the size of deformed ellipse is different at different locations。
2) Equal product Projection
It is defined that a certain differential area remains equal before and after projection, that is, its area ratio is 1, that is, any area on the projection plane is equal to the corresponding area on the ellipsoid, that is, the area deformation is equal to zero。
3) Isometric Projection
In any projection, the length, area and angle are deformed, which is neither equal nor equal. But in arbitrary projection, there is a common equidistant projection, which is defined as the distance along a specific direction, and the projection remains unchanged before and after, that is, the length ratio along that particular direction is 1. In this projection map, there is no length distortion, but there is no length distortion in a specific direction. The area distortion of isometric projection is less than that of isometric projection, and the angle distortion is less than that of isometric projection. Arbitrary projection is mostly used for maps that require small area deformation and small angle deformation, such as general reference maps and teaching maps. After projection, the length, area and angle deformations on the map are interrelated and interacted. The relations between them are as follows: the equiangular property can not be maintained in the equiangular projection, the equiangular property can not be maintained in the equiangular projection; the equiangular and equiangular property can not be maintained in the arbitrary projection; the shape distortion of the equiangular projection is relatively large, and the area distortion of the equiangular projection is relatively large。
Classified according to the method of composition
Map projection was originally based on the geometric principle of perspective. It directly penetrates the ellipsoid into a plane or onto a developable surface, such as a cylinder and a cone. Cylindrical and conical surfaces are not planar, but they can be expanded into planes. In this way, geometrically meaningful azimuth, cylindrical and conical projections can be obtained. With the development of science, in order to minimize the deformation on the map, or to satisfy some specific requirements, map projection gradually jumps out of the original frame of projection by means of geometric surface, and produces a series of projections according to mathematical conditions. Therefore, according to the method of composition, map projection can be divided into two categories: geometric projection and non-geometric projection。
1) Geometric Projection
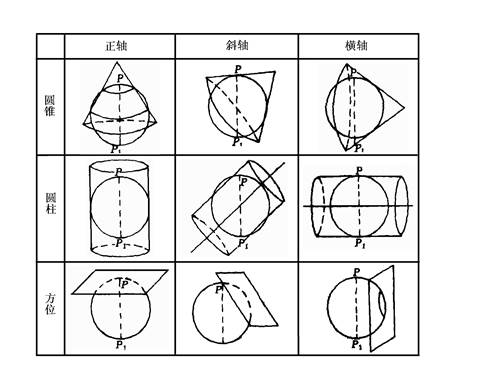
Geometric projection is obtained by projecting the longitude and latitude lines mesh on the ellipsoid to the geometric surface, and then the geometric surface is expanded into a plane. According to the shape of the geometric surface, it can be further divided into the following categories (Fig. 4-11):
(1.1) ** Azimuth projection** A plane is used as a projection surface, so that the plane and the sphere are tangent or cut, and the longitude and latitude lines on the sphere are projected onto the plane。
(1.2) **Cylinder projection** The cylindrical surface is used as the projection surface to make the cylindrical surface tangent or cut with the sphere. The longitude and latitude lines on the sphere are projected onto the cylindrical surface, and then the cylindrical surface is expanded into a plane。
(1.3) **Cone projection** The conical surface is used as the projection surface, so that the conical surface and the sphere are tangent or cut, the longitude and latitude lines on the sphere are projected to the conical surface, and then the conical surface is expanded into a plane. Here, we can consider azimuthal projection as a special case of conical projection, assuming that when the apex angle of the cone is expanded to 180 degrees, the conical surface becomes a plane, and then the longitude and latitude lines on the ellipsoid of the earth are projected onto the plane. Cylindrical projection, by geometrical definition, is also a special case of conical projection. It is assumed that when the conical vertex extends to infinity, it will become a cylinder。
2) Non-geometric Projection
Without the help of geometric surface, the function relation between point and point between sphere and plane is determined by mathematical analytic method according to some conditions. In this kind of projection, it is generally divided into the following categories according to the shape of longitude and latitude:
Pseudo-azimuth Projection : The latitude is a concentric circle, the central longitude is a straight line, and the rest of the longitude are symmetrical to the central longitude curve, and intersect at the common center of the latitude。
Pseudocylindrical projection : The latitude is a parallel line, the central longitude is a straight line, the rest of the longitude are symmetrical to the central longitude curve。
Pseudo-conical Projection : The latitude is a concentric arc, the central longitude is a straight line, and the other longitudes are symmetrical to the central longitude。
Multi-conical projection : The latitude is an arc of the same circumference. The center of the circle is on the central meridian, the central meridian is a straight line, and the other meridians are symmetrical to the central meridian。
Classification by projection area cut or tangent to the earth
1) Cut Projection
The plane, cylindrical or conical surface is used as projection surface to cut the projection surface from the sphere. Longitudinal and latitudinal lines on the sphere are projected onto the plane, cylindrical or conical surface, and then the projection surface is expanded into a plane。
2) Cut projection
Plane, cylindrical or conical surface is used as projection surface, and the projection surface is tangent to the sphere. Longitudinal and latitudinal lines on the sphere are projected onto the plane, cylindrical or conical surface, and then the projection surface is expanded into a plane。
Selection of Map Projection
Whether the map projection is selected properly or not directly affects the accuracy and value of the map. The selection of map projection here mainly refers to medium and small scale maps, excluding national basic scale topographic maps. Because the projection and division of national basic scale topographic maps are studied and formulated by the competent national surveying and mapping authorities[1]_, it is not allowed to change arbitrary,and large-scale maps of small areas are compiled. No matter what projection is used, the deformation is very small。
When choosing cartographic projection, the following factors should be considered: the scope, shape and geographical location of the cartographic area, the use of maps, publishing methods and other special requirements, among which the scope, shape and geographical location of the cartographic area are the main factors。
For the world map, the main ones are the positive cylindrical, the pseudo-cylindrical and the multi-conical projections. In the world map, the Mercator projection is commonly used to draw the world route map, world traffic maps and world time zones;
Most of the world maps published in China are projected by equal difference latitudes and cones. This projection is good for representing the shape of China and the comparison with its neighbors, but the edge of projection deforms a lot。
For hemispheric maps, the horizontal axis azimuth projection is often used in the eastern and Western Hemisphere maps, the normal axis azimuth projection is often used in the southern and Northern Hemisphere maps, and the oblique axis azimuth projection is generally used in the water and land hemisphere maps。
For other medium and small range projection selection, the contour shape and geographical location should be taken into account. It is better to make the contour shape of the isomorphic line basically consistent with the contour shape of the mapping area, so as to reduce the deformation on the map. Therefore, Therefore, the circular area is generally suitable for azimuthal projection, the orthogonal azimuth projection near the poles, the transverse azimuth projection in the equator-centered areas and the oblique azimuth projection in the mid-latitude areas. In the mid-latitudes extending from east to west, the orthogonal conical projection is usually used, such as China and the United States. Orthogonal cylindrical projection, such as Indonesia, should be used in areas extending East-West on both sides of the equator. Transverse cylindrical projection and multi-conical projection, such as Chile and Argentina, are commonly used in areas extending in the North-South direction。
Some commonly used map proojections
Projection of the World Map
The projection of the world map mainly considers to ensure that the global deformation is not large. According to different requirements, it needs to have equiangular or iso-product properties, mainly including: iso-differential latitude multi-conical projection, tangent difference latitude multi-conical projection (1976 plan), arbitrary pseudo-cylindrical projection, orthogonal equiangular secant cylindrical projection。
Projection of a hemispheric map
The eastern and western hemispheres have an azimuthal projection of the horizontal axis and an isometric projection of the horizontal axis. The southern and northern hemispheres have equal area azimuthal projection, equal angle azimuthal projection and equal distance azimuthal projection。
Map projection of continents
Projection of Asian maps: oblique equi-area azimuth projection and Penner projection。
Projection of the European maps: oblique equal-area azimuth projection and orthogonal equal-angle conical projection。
Map projection of North America: oblique equi-area azimuthal projection and Penner projection。
Map projection of South America map: oblique equal-area azimuth projection and Thomson projection。
Projection of Australian maps: oblique equal-area azimuthal projection and orthogonal equal-angle conical projection。
Projection of a map of Latin American: oblique equi-area azimuthal projection。
Various map projection in China
Map projection in China: oblique equal-area azimuth projection, oblique equal-angle azimuth projection, Penner projection, pseudo-azimuth projection, orthogonal equal-area secant conical projection and orthogonal equal-angle secant conical projection。
Projection of maps of provincial (regional) in China: equiangular secant conical projection, equiangular secant conical projection, equiangular cylindrical projection and Gauss-Kruger projection (broadband)。
Projection of China’s large-scale map: polyhedron projection (during the period of the Northern Warlords), equiangular secant conical projection (Lambert projection) (before liberation), Gauss-Kruger projection (after liberation)。