1. Home
  2. App
  3. Trigonometric
  4. Text

The second normal total variance is compared to the hypothesis test _ online calculation tool

Category: Trigonometric
Help edit

1,Enter sample values or sample size and calculated sample mean and sample standard deviation:
Enter each sample value Enter sample size, sample mean, and sample standard deviation
Enter the individual sample values for the general X and Y in the following multi-line input box, and the sample values for X and Y are separated by the character "|", and the individual sample values are separated by a comma or blank characters
2,Select the significance level α：
0.1 0.05 0.025 0.02 0.01 0.005 0.001
3,3,Set the hypothesis option (original hypothesis is ): H0 ,alternative hypothesis is H1)：
H0:σ1 2=σ2 2, H1:σ1 2≠σ2 2
H0:σ1 2≤σ2 2, H1:σ1 2>σ2 2
H0:σ1 2≥σ2 2, H1:σ1 2<σ2 2
4,Click the "Start Inspection" button to start checking:

App description

Suppose the two populations X and Y are independent of each other and obey the normal distribution. The mean and variance of X areμ1,σ12, the mean and variance of Y areμ2,σ22, that is X~N(μ1,σ12), Y~N (μ2,σ22),this page is the differenceσ12/σ22 hypothesis testing, the statistics are

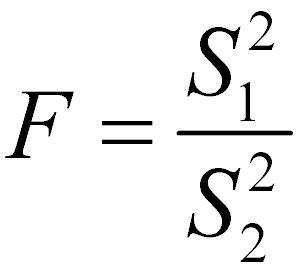
，S12,S22For the sample variance of the two sets of samples, their square root is called the sample standard deviation, n1, and n2 is the corresponding sample size.

 

    Sign in for comments!
Submit
Comment list (0)

Label:
Evaluation
Related app
App classification
Recent used
Most use

Powered by TorCMS (https://github.com/bukun/TorCMS).